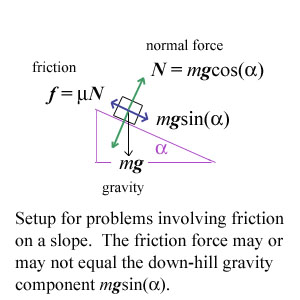
Most of these problems are friction problems. For friction on a slope, draw this diagram:

FIG 1

Always decompose mg into its down-slope and into-slope components. The normal force must be balance the into-slope component, in order to prevent the object from sinking into the ramp. If the object is stationary or slides without acceleration, then the friction force f must balance the down-slope component of gravity. If you do the math in that case, you find that the coefficient of friction equals the tangent of the angle.

For friction on a level surface, draw this diagram:

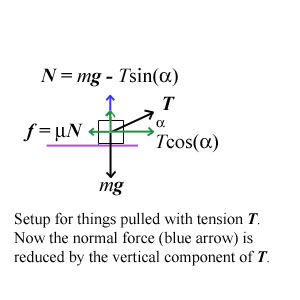
FIG 2

Here the tension (if there is any) helps relieve the "need" for the surface to push up on the object, so the normal force is reduced (and along with it the friction).

1: Both blocks accelerate as if they were one block of 15 kg. Therefore the acceleration is a=F/m=2 m/s². The second block is accelerating at this value, so the force on it must be F=ma=12 N. That is the tension.

2: We could make a equivalent horizontal problem. Two blocks are connected with a string; one has a force 10g N to the left, the other a force 2g N to the right. Now, both blocks behave as one, so the net force is 8g to the left, acting on 12 kg of mass. So the acceleration will be a=8g/12=6.5 m/s²,

4. For the trapeze artist, the vertical component of T must = 800 N, and the horizontal component is balanced by the partner. The horizontal / vertical components have the ratio 1/sqrt(3), so they are 462 and 800 N respectively. T itself becomes 924 N.

6. For the tightrope walker, the vertical component of the tension on each half rope must be 250 N to match the weight of the walker. Thus each rope half must be pulling at 250/sin(10deg) N. Since this force is the force at the end of the rope, it is the tension.

8. This problem is a "Figure 2" problem. Since there is no acceleration, the friction force must be balancing the horizontal component of the tension.

9. Here we determine the acceleration from x=at²/2 and v=at [so x=v²/(2a)]. Then the friction force is ma, so the coefficient of friction is a/g.

After chapter 5, we could solve this one more easily with W=Fx=mv²/2.

10. This is a "Figure 1" problem. The answer is the angle the tangent of which is 0.77.

11. This one requires two drawings, one for each "weight". For the 9kg weight, we have m₉a=m₉g-T, while for the 5kg weight we have m₅a=T-(mu)*m₅g. We can solve these two equations in two unknowns for T and a (the accelerations of both weights are the same).